2D Patterns

Prerequisites

This mini-project draws on materials from:

• Shapes and Colors
• Drawing Patterns
• Loops as Counters
• Translations and Transformations

Tiling

We saw how to draw chessboards here.

What if instead of black and white squares we used the following tiles:

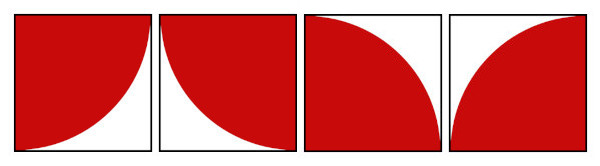
The function quarter() in the sketch below uses four parameters (x, y, width and angle) to draw a tile at position (x, y) that has a quarter-circle in one of its corners.

That is cool, and we can experiment with the angles in this version and use \(0\) and \(180\), but this code will only use \(2\) out of many possible different variations of the pattern.

We can try something that rotates depending on all possible row and column even/odd combinations:

if (col is even && row is even) {
  angle = 0;
} else if (col is even && row is odd) {
  angle = 90;
} else if (col is odd && row is even) {
  angle = 180;
} else if (col is odd && row is odd) {
  angle = 270;
}

Or, many other possible combinations of the \(4\) angles.

Try to change the order of the angles to \([0, 180, 90, 270]\) or \([0, 270, 180, 90]\).

Or, use repeated angles: \([90, 180, 90, 270]\) or \([0, 180, 180, 90]\).

Cool, right?!

One more

Let’s use a pattern like this now:

It’s made up of a single composite shape, but depending on which column or row it’s in, it gets rotated by \(0^\circ\), \(90^\circ\), \(180^\circ\) or \(270^\circ\).

Without worrying too much about the implementation details, let’s say we have a function called zig() that draws that shape based on \(4\) parameters: x, y, width and angle of rotation (in degrees):

Again, we can just plug that into our previous chessboard sketch and instead of alternating colors, alternate angle:

And, again, using all \(4\) possible combinations of row/column even/odd:

Change the order of the angles: like \([0, 180, 90, 270]\) and \([0, 270, 180, 90]\), or, use repeated angles: \([90, 180, 90, 270]\) and \([0, 180, 180, 90]\).